チェビシェフの多項式


\(\cos \) で成り立つ性質ということは,\(\sin \) でも成り立ちますか??

残念ながら,\(\sin\) ではうまくいきません!
ここでは説明は省略しますが,\(\sin n \theta\) は\(\sin \theta\) と\(\cos \theta\) の \(n-1\) 次多項式の積で表されることがわかっています!
具体例(\(n=1,2,3,4\) )

確かに\(\cos n \theta\) は, \(\cos \theta\) の \(n\) 次多項式で表せそうですね!
さらに,最高次の係数は,\(2^{n-1}\) になることも予想できます!
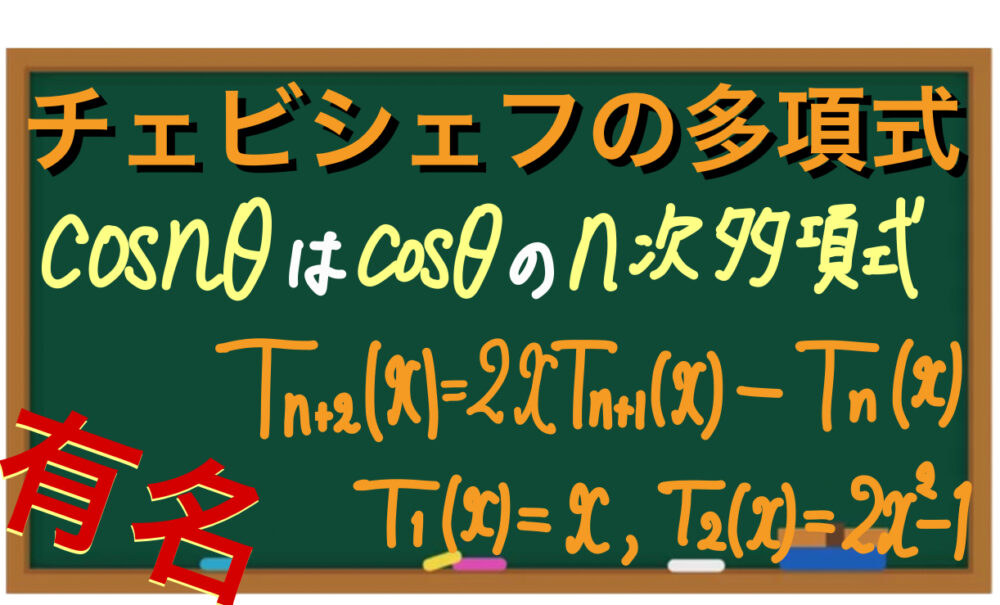
チェビシェフの多項式と漸化式
すべての自然数 \(n\) に対して,\(\cos n \theta=T_{n}(\cos \theta)\) で表せる.
\(T_{1}(x)=x\) ,\(T_{2}(x)=2x^2-1\)
\(T_{n+2}(x)=2xT_{n+1}(x)-T_{n}(x)\)

下記でこの三項間の漸化式が成立することを証明しましょう!
チェビシェフの多項式(証明)
\(n=1,2\) のとき,\(T_{1}(x)=x\) ,\(T_{2}(x)=2x^2-1\) が成立することは明らか.
三角関数の加法定理より
\(\cos(n+1) \theta=\cos n \theta\cos \theta-\sin n \theta\sin \theta\)
\(\cos(n-1) \theta=\cos n \theta\cos \theta+\sin n \theta\sin \theta\)
\(2\) 式を加えると
\(\cos(n+1) \theta+\cos(n-1) \theta=2\cos n \theta\cos \theta\)
\(\cos(n+1) \theta=2\cos n \theta\cos \theta-\cos(n-1) \theta\)
よって,
\(\cos(n+2) \theta=2\cos \theta\cos(n+1) \theta-\cos n \theta\)
であるから,
\(T_{n+2}(x)=2xT_{n+1}(x)-T_{n}(x)\) が成立.






コメント